The Persistence of Memory by Serkan Yürekli
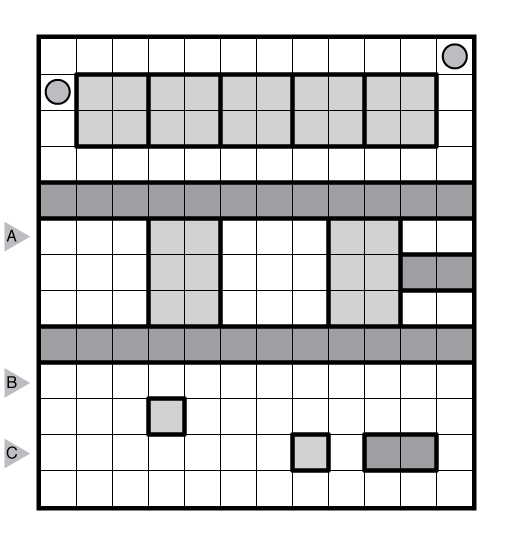
or solve online (using our beta test of Penpa-Edit tools using a composite mode where left click inside cell shades square, left click + drag draws line segment, right click inside cell adds dot, and right click on cell edge adds an x.)
Theme: Quadrilaterals
Author/Opus: This is the 92nd puzzle from our contributing puzzlemaster Serkan Yürekli.
Rules: Draw a snake (a 1-cell wide path) from one dot to the other by moving horizontally or vertically between adjacent squares. The snake cannot touch itself, not even diagonally. All highlighted regions must be visited by the snake, and may be re-entered. If two or more highlighted regions have the same shape and orientation, then how the path passes through those shapes must be identical.
Answer String: Enter the number of cells used by each snake segment from left to right for the marked rows, starting at the top. Use both digits for any two-digit number. Separate each row’s entry with a comma.
Time Standards (highlight to view): Grandmaster = 2:00, Master = 3:45, Expert = 7:30
Solution: PDF
Note: Follow this link for other Snake puzzles.

Coming back much later while inputting solutions, I was fairly shocked to find no comments on this one.. Really enjoyed the logical solve path.
Question about the rules, “how the path passes through those shapes must be identical.” Does this include which cell boundaries are passed through (entering/exiting the regions), or only which cells within the regions?
This is an exact matching of the paths, so it includes the same cells used, as well as the same cell boundaries passed through in each region.
I am totally stumped by this puzzle. By the rules as I understand them, I can prove that it is unsolvable. So, either my understanding of the rules is wrong, or my proof is wrong. I’ve reviewed both a half dozen times, and I can’t find my error. Is there another example of this puzzle type on this website?
This is probably just a misunderstanding of the rules. Here are other instances of the Persistence of Memory on this site. This example from Serkan may also help you.
(Note: I rewrote the instructions for this week as it was all Snake puzzles, but perhaps the instructions I used on the earlier posts will be more clear.)
Aha! Thanks, the example cleared everything up.
6:27.Nice