Round Trip by Bryce Herdt
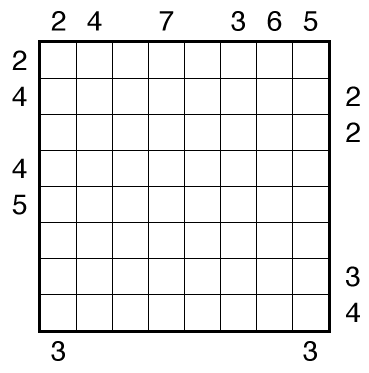
or solve online (using our beta test of Penpa-Edit tools; in default linex mode: left-click+drag draws line, right click marks X on edge)
Theme: 24-7-365
Author/Opus: This is the 2nd puzzle from guest contributor Bryce Herdt (the 1st was his winning submission for our first hidden contest).
Rules: Draw a single loop in the grid which may cross itself orthogonally, but otherwise does not touch or retrace itself. The clue numbers to the left/right of the rows indicate the number of squares visited by the nearest section of the loop that travels horizontally in the rows. The clue numbers to the top/bottom of the columns indicate the number of squares visited by the nearest section of the loop that travels vertically in the columns.
Also, see this example:
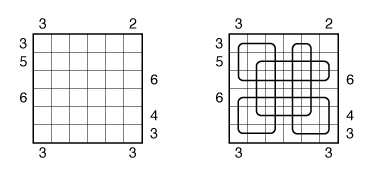
Answer String: Enter the number of empty squares for each row from top to bottom, followed by a comma, and then the number of turns for each row from top to bottom. This example has the key “100000,422224”.
Time Standards (highlight to view): Grandmaster = 3:45, Master = 6:45, Expert = 13:30
Solution: PDF
Note: Follow this link for other Round Trip puzzles. Some more Round Trip puzzles can be found in the book Loop Variety Collection by Ashish Kumar and Murat Can Tonta.

Oy…having done some Castle Walls this week, I kept trying to get the loop _lengths_ to match the numbers of the side, rather than the number of cells.
Sorry about that. It’s a known pitfall I haven’t figured out a good way to avoid when posting these. I’m probably hesitating too much to change Craig’s original puzzle definition to bring it in line with how loop counting happens for all other genres on the site.
It’s an interesting question. When I first started solving loop/path puzzles here, the counting seemed counter-intuitive to me. But for most styles it was only for entering soluting strings, so it wasn’t a big deal. When I first encountered Castle Wall, (where loop lengths are used while solving), I found the hint in the rules about counting cell-border crossings very helpful. Then, by the time Balance Loop was introduced I realized that the counting convention already felt natural to me.
So the puzzles did win me over to the approach, even though it felt strange to me at first to not count cells.
Here’s one rationale you might consider for changing the convention of Round Trip. In addition to consistency with the other puzzle genres, it would also mean that the number 1 would then be available for puzzle authors, and that might help for theme possibilities.
I have to say, I fell into the same trap and had to start over when I realised the rightmost column was impossible under that scheme. I have to agree with Carl on this too, while that method of counting loop lengths felt counter-intuitive at first it now seems odd to not use it here.
Would changing it mean redoing the clues on all the prior round trips though?
As someone who has come in late (so to speak) and then gone back to solve some of the older puzzles, I would have found it really confusing if some puzzles of the same type used a slightly different rule set.
That said, my main issue with this puzzle type is still the answer string format which I still find… awkward.
– Neelix
I would indeed go back and change all the puzzles we’ve posted (6?) to use one less on clues.
I’d probably only change the answer entry going forward though so that I don’t need to reset all those strings (which will also reset the “solved” status for the puzzle). For answer entry, would you prefer a “S = Straight, T = Turn, X = crossover, B = blank” system for some marked rows so something like “TSXTBBTT,…”?
@drsudoku
re: STXB notation; Yeah, I think that could work well. 🙂
– Neelix
What about L for turns and I for straights?
Perhaps I should chime in here.
When I created this type, the line of experimentation that it came from was exploring what a “Cross the Streams”-type puzzle might look like as a path/loop puzzle. Accordingly, I kept the CtS clue notation (numbers are numbers of squares) without even considering the alternative of using center-point distance/numbers of wall crossings instead.
I have no objection in principle to making the change, though there are practical details that would need to be worked out, of course.
Lastly, I must say that I’m gratified that this puzzle type has caught on with other puzzle constructors, and I’m always pleased to see additional examples of the puzzle type – and to solve them. 🙂
Thanks for sharing some of the puzzle history, Carig.
It occurs to me that one could make Round Trip even more like Cross the Streams by using sequences of clues on the left and the top. (So for, example, take any of the existing puzzles and replace each existing clue with either “X *”, “* Y”, or “X Y” as appropriate, and similarly for top and bottom.)
That wouldn’t add anything to any of the current puzzles, (and admittedly, would make the presentation less elegant). But it might be something interesting to consider to allow the puzzle to scale up to larger sizes, (where the current numbers only “seeing” the nearest segment could make it difficult to make puzzles unique at large sizes).
You get used to either way quickly in puzzling. There’s a lot of different uses of similar clue types. It’s no different from two Sudoku types both using shaded cells to indicate regions (say Clone and Renban Sudoku).
For me the counting in Cave/Corral puzzles felt very unnatural for a long time, but I have gotten used to it. This is because I started solving Seethrough(also known as Open Office and others) and Four Winds puzzles, which don’t count the cell itself. Nowadays I can do both ways easily without breaking me up.
The real trick in competition is to not solve them too closely together, but to give yourself time to get rid of the previous thought process. I always hate solving Anti-Knight and Non-Consecutive Sudokus in consecutive solves for example, because the similar layout makes my brain try to make deductions from the previous Sudoku variant in the second solve.
Phew that was really hard for me. Not sure what the strictly logical path was, it felt like i was guessing very much to find contradictions.. i happen to like the square counting system, i think that it is much more intuitive and natural than the loop segments. Even after solving some loop puzzles here i get confused every time i have to enter an answer string.. i always see the number of squares first and then have to subtract one, especially if there are long segments involved.
It does make sense as a way of counting the length of a line that travels within a row. Otherwise a line that just crosses the row counts as one.
The thing to do is focus on the lines it crosses, rather than the squares. I had the same problem you describe until I realised this trick.
I found this especially useful for Balance Loop, as you don’t have to worry about discounting the numbered square.
– Neelix
26:05 A great challenge in this Round Trip, thanks!
It’s funny that even after participating in the discussion above about how to treat the numbers in Round Trip, when I went to solve this I first started using the wrong counting. Fortunately, I hit a contradiction very early that way.
I’d be curious to know if the difficulty of Round Trip puzzles scales with the number of crossings in the solution. Intuitively, it seems so, (or maybe I just keep making mistakes by not considerign crossings when I should).
About halfway through I started tweaking my notation by only drawing partial paths just across the cell borders, (and not all the way to the center). I think that should help me avoid looking at a half-drawn crossing and thinking that it’s a deduced turn in the path.
I do the exact opposite — I extend both segment beyond the crossing point, so that, to my eye, the crossing looks incomplete. Then I erase the superfluous segments if it turns out it really is a turn.
24:56.Nice